SimplicityTheory

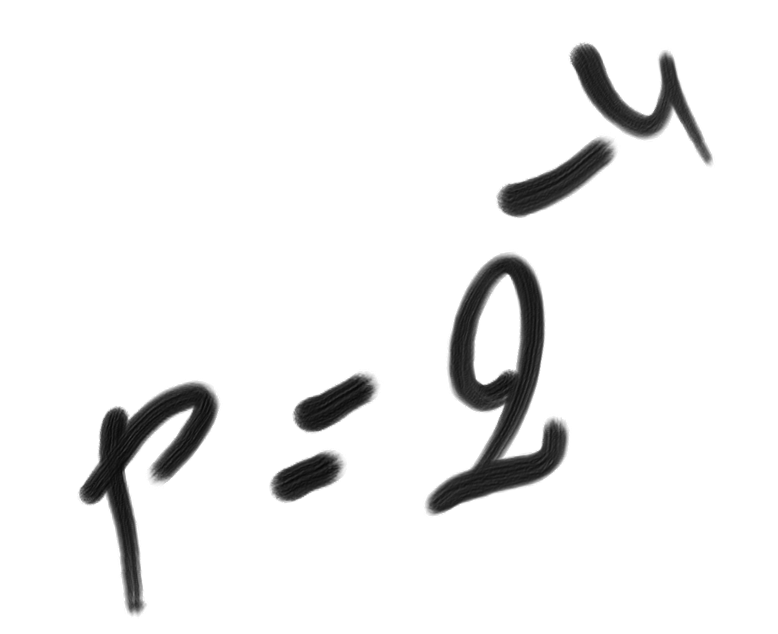Simplicity, Complexity, Unexpectedness, Cognition, Probability, Information


by Jean-Louis Dessalles     (created 31 December 2008, updated March 2020)

The "next door" effect (proximity)

Closer events are simpler and therefore more unexpected.
The person who yawns over a report that famine has swept a million Chinese to their graves will snap to attention if he learns his neighbor’s child is in the hospital. And if his own child is hospitalised or, say, wins a prize in school, causing the family name to appear in the paper, that item -from his viewpoint- is packed with interest. (Warren 1934/1959:21)

News is a perishable product, good only when fresh. (Warren 1934/1959:15)

    

In November 2007, as I headed toward the Centro di Scienza Cognitiva in Torino to give a lecture about unexpectedness, I witnessed an accident between a car and a tram just one block away from via Po. I took the photo and made some impression by showing it during the talk, as I was speaking about the importance of space and time proximity.

Why are events more unexpected when they happen closer? And how much more?
By definition, unexpectedness U is the difference between generation complexity and description complexity: Cw – C.

    

Generation complexity Cw

Suppose you know from experience that the kind of event s you consider (e.g. an accident involving a tram) occurs with spatiotemporal density De , which means De = 1/Ve if there is one occurrence on average per spatiotemporal volume Ve.

Notes:

  1. You may estimate De by making Ve equal to the smallest hypervolume centred on self enclosing the closest remembered instance of s; it can be shown to be a good estimator of De. In other terms, you may retrieve from memory the latest and closest occurrence of s.
  2. Relevant dimensions include space and time, but also social distance. Social distance should be taken as proportional to gk where k is the distance in the acquaintance graph and g the average degree in this graph (supposing k remains small to avoid small-world effects).
Suppose event s has a spatiotemporal volume vs (to locate an event like a tram accident, we are dealing with a few hundred square meters within a few minutes). To generate the location of the event, the "world-machine" must decide among Ve/vs possibilities. The generation complexity amounts therefore to: $$C_w(s) = \log_2 (\frac{V_e}{v_s})$$

Description complexity C

When the event is close, its location requires less complexity. Let’s consider first two spatial dimensions only. Suppose locations are ranked egocentrically, by increasing distance from self. There are about v_sub(s)) --> \( 2 \pi d / \sqrt{v_s} \) different locations at distance d (the approximation is good for \(d / \sqrt{v_s}\) not too small). A location x at distance d in a two-dimension space requires no more than \(\log_2(\pi d^2/v_s)\) bits to be unambiguously determined: C(x) < log2(πd2/vs)
More generally, if the event occurs within an ego-centred spatiotemporal volume ve, then the complexity of x amounts to: $$C(x) \le \log_2 (\frac{v_e}{v_s})$$ Finally: $$\boxed{U(x) \ge \log_2 (\frac{V_e}{v_e})}$$ This explains why close events (ve small) are more unexpected.

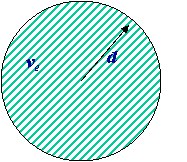If we consider only spatial dimensions in a two-dimension space, then:

U(l) > 2 × log2 (R/d)

where d is the distance to the event and R is the distance to the closest remembered occurrence.

In a one-dimension space, we would have: U(x) > log2 (R/d). This accounts for recency effects.

When social distance is taken into account, U is proportional to the degree of separation in the social graph.

Bibliography

Dessalles, J.-L. (2007). Spontaneous assessment of complexity in the selection of events. Technical Report ParisTech-ENST 2007D011.

Dessalles, J-L. (2008). Coincidences and the encounter problem: A formal account. In B. C. Love, K. McRae & V. M. Sloutsky (Eds.), Proceedings of the 30th Annual Conference of the Cognitive Science Society, 2134-2139. Austin, TX: Cognitive Science Society.

Dessalles, J-L. (2008). La pertinence et ses origines cognitives - Nouvelles théories. Paris: Hermes-Science Publications.

Dimulescu, A. & Dessalles, J-L. (2009). Understanding narrative interest: Some evidence on the role of unexpectedness. In N. A. Taatgen & H. van Rijn (Eds.), Proceedings of the 31st Annual Conference of the Cognitive Science Society, 1734-1739. Amsterdam, NL: Cognitive Science Society.

Warren, C. N. (1934). Modern news reporting. New York: Harper & Brothers, ed. 1959.

Back to the Simplicity Theory page.